
Trial function for the quantum double-well potential
Date: 2015-10-07; view: 733.
To illustrate how to construct a trial function, consider the quartic potential in one dimension with degenerate minima:
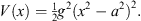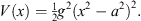
| (2.29) |
An alternative form of the same problem can be obtained by setting 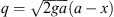 so that the Hamiltonian becomes
so that the Hamiltonian becomes
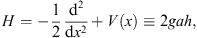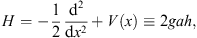
| (2.30) |
where
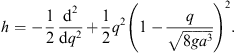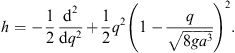
| (2.31) |
This shows that the dimensionless (small) expansion parameter is related to  ; as it turns out, the relevant parameter is its square. In the following, we shall take a = 1 so that the expansion parameter is 1/g; in the literature [5], [6], [7], [8], [9], [10], [11], [12], [13] and [14] one often finds the assumption 2ga = 1 (placing the second minimum of the potential at q = 1/g) so that
; as it turns out, the relevant parameter is its square. In the following, we shall take a = 1 so that the expansion parameter is 1/g; in the literature [5], [6], [7], [8], [9], [10], [11], [12], [13] and [14] one often finds the assumption 2ga = 1 (placing the second minimum of the potential at q = 1/g) so that  reduces to g and the anharmonic potential appears as (1/2)q2 (1−gq)2. Then g appears with positive powers instead of negative, but the coefficients of the power series are the same as with our form of the potential, apart from the overall factor 2ga.
reduces to g and the anharmonic potential appears as (1/2)q2 (1−gq)2. Then g appears with positive powers instead of negative, but the coefficients of the power series are the same as with our form of the potential, apart from the overall factor 2ga.
For the above potential (2.29), the Schroedinger equation (2.4) is (with a = 1)
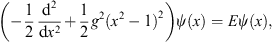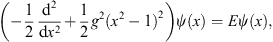
| (2.32) |
where, as before, ψ (x) = e−gS (x) is the ground state wave function and E its energy. Using the expansions Figs. (2.6) and (2.7) and following the steps Figs. (2.8) and (2.10), and Figs. (2.15), (2.16), (2.17), (2.18), (2.19), (2.20) and (2.21), we find the well-known perturbative series

| (2.33) |
and
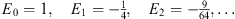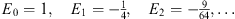
| (2.34) |
Both expansions S = S0 + g−1S1 + g−2S2 +  and E = gE0 + E1 + g−1E2 +
and E = gE0 + E1 + g−1E2 +  are divergent, furthermore, at x = −1 and for n
are divergent, furthermore, at x = −1 and for n  1, each Sn (x) is infinite. The reflection x → −x gives a corresponding asymptotic expansion Sn (x) → Sn (−x), in which each Sn (−x) is regular at x = −1, but singular at x = +1. We note that for g large, the first few terms of the perturbative series (with (2.33) for x positive and the corresponding expansion Sn (x) → Sn (−x) for x negative) give a fairly good description of the true wave function ψ (x) whenever ψ (x) is large (i.e., for x near ±1). However, for x near zero, when ψ (x) is exponentially small, the perturbative series becomes totally unreliable. This suggests the use of first few terms of the perturbative series for regions whenever ψ (x) is expected to be large. In regions where ψ (x) is exponentially small, simple interpolations by hand may already be adequate for a trial function, as we shall see. Since the quartic potential (2.29) is even in x, so is the ground state wave function; likewise, we require the trial function
1, each Sn (x) is infinite. The reflection x → −x gives a corresponding asymptotic expansion Sn (x) → Sn (−x), in which each Sn (−x) is regular at x = −1, but singular at x = +1. We note that for g large, the first few terms of the perturbative series (with (2.33) for x positive and the corresponding expansion Sn (x) → Sn (−x) for x negative) give a fairly good description of the true wave function ψ (x) whenever ψ (x) is large (i.e., for x near ±1). However, for x near zero, when ψ (x) is exponentially small, the perturbative series becomes totally unreliable. This suggests the use of first few terms of the perturbative series for regions whenever ψ (x) is expected to be large. In regions where ψ (x) is exponentially small, simple interpolations by hand may already be adequate for a trial function, as we shall see. Since the quartic potential (2.29) is even in x, so is the ground state wave function; likewise, we require the trial function  (x) also to satisfy
(x) also to satisfy  (x) =
(x) =  (−x). At x = 0, we require
(−x). At x = 0, we require
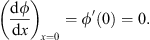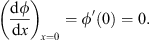
| (2.35) |
To construct  (x), we start with the first two functions S0 (x) and S1 (x) in (2.33). Introduce, for x
(x), we start with the first two functions S0 (x) and S1 (x) in (2.33). Introduce, for x  0,
0,
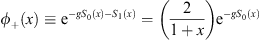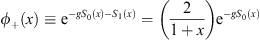
| (2.36) |
and
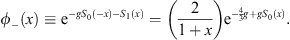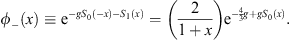
| (2.37) |
In order to satisfy (2.35), we define
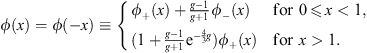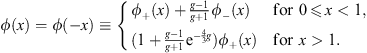
| (2.38) |
Thus, by construct  ′ (0) = 0,
′ (0) = 0,  (x) is continuous everywhere, for x from −∞ to ∞, and so is its derivative.
(x) is continuous everywhere, for x from −∞ to ∞, and so is its derivative.
By differentiating  + (x) and
+ (x) and  (x), we see that they satisfy
(x), we see that they satisfy
(T+V+u)  +=g +=g  + +
| (2.39) |
and
(T+V+w)  =g =g  , ,
| (2.40) |
where
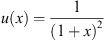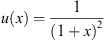
| (2.41) |
and
| w(x)=w(-x) | (2.42) |
with, for x  0
0
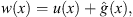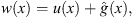
| (2.43) |
where

| (2.44) |
Note that for g > 1, gˆ (x) is positive, and has a discontinuity at x = 1. Furthermore, for x positive both u (x) and gˆ (x) are decreasing functions of x. Therefore, w (x) also satisfies for x > 0,
| w′(x)<0, | (2.45) |
a property that is very useful in our proof of convergence which will be discussed in the next section.
| <== previous lecture | | | next lecture ==> |
| A new formulation of perturbative expansion | | | Hierarchy theorem and its generalization |