The median

Date: 2015-10-07; view: 337.

The median is the middle term in a data set. Before one can find this point the data must be arranged in increasing (or decreasing) order. The calculation of the median for ungrouped data consists of the following two steps:

1. Rank the given data set in increasing (or decreasing) order.

2. Find term in a ranked data set.

The value of term is the median.

There are two possibilities

1) If 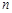is odd, then the median is given by the value of the middle term in a ranked data.

2) If 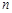is even, then the median is given by the average of the values of the two middle term.

Remark: If the given data set represents a population, replace 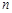by .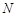

Example:

Consider again the seven salaries of employees of a small company

$ 320, 410, 310, 480, 530, 370, 240

Calculate the median of this population.

Solution:

First of all, let us rank salaries in ascending order:

$ 240, 310, 320, 370, 410, 480, 530

N=7 and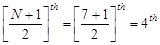

Therefore, the median is the value of the fourth term in the ranked data

$ 240, 310, 320, 370 , 410, 480, 530

Thus, the median value for this population is $370.

Example:

The ages of a sample of 10 university students are

18, 22, 19, 20, 21, 18, 22, 19, 23, 17

Calculate the median of this sample.

Solution:

First we order the data in increasing order. The ordered vales are

17, 18, 18, 19, 19, 20, 21, 22, 22, 23

There are 10 values in the data set. Hence,

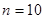and

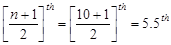
Therefore, the median is given by the mean of fifth and sixth values in the ranked data.

,

.

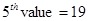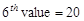
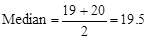Hence, the median age is 19.5.